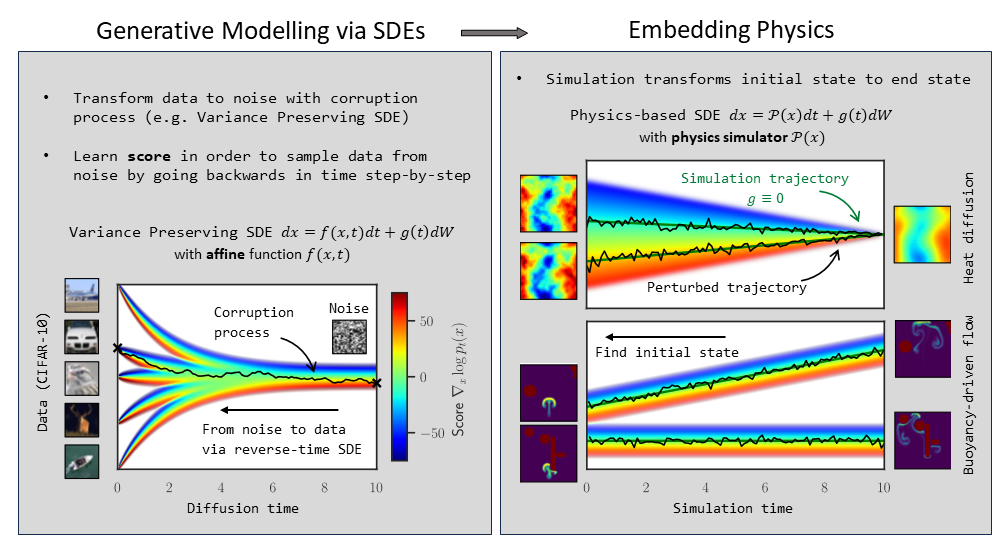
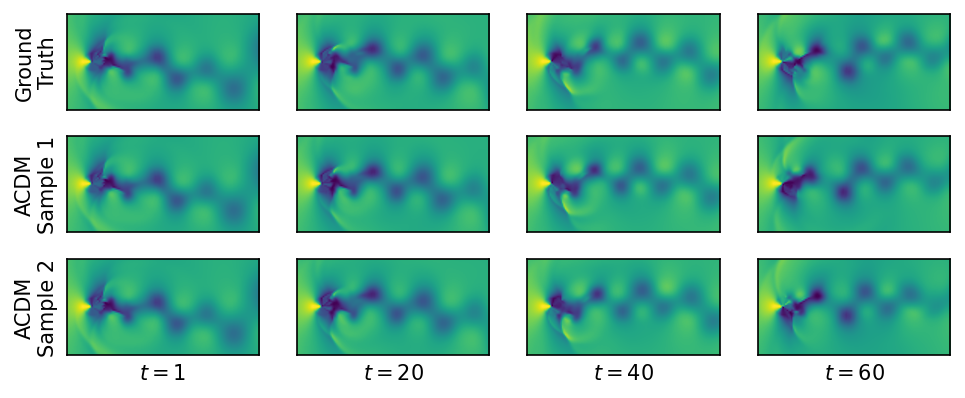
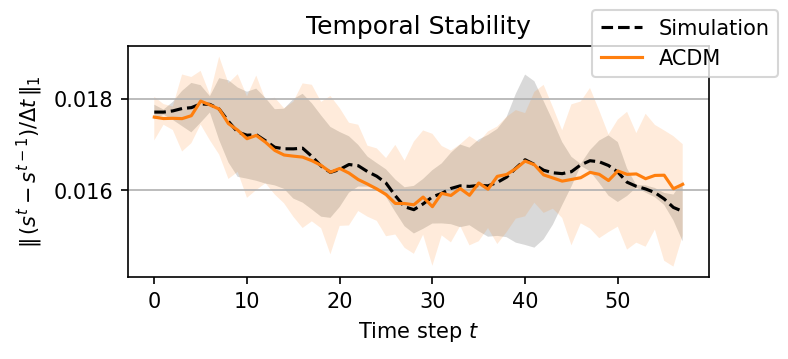
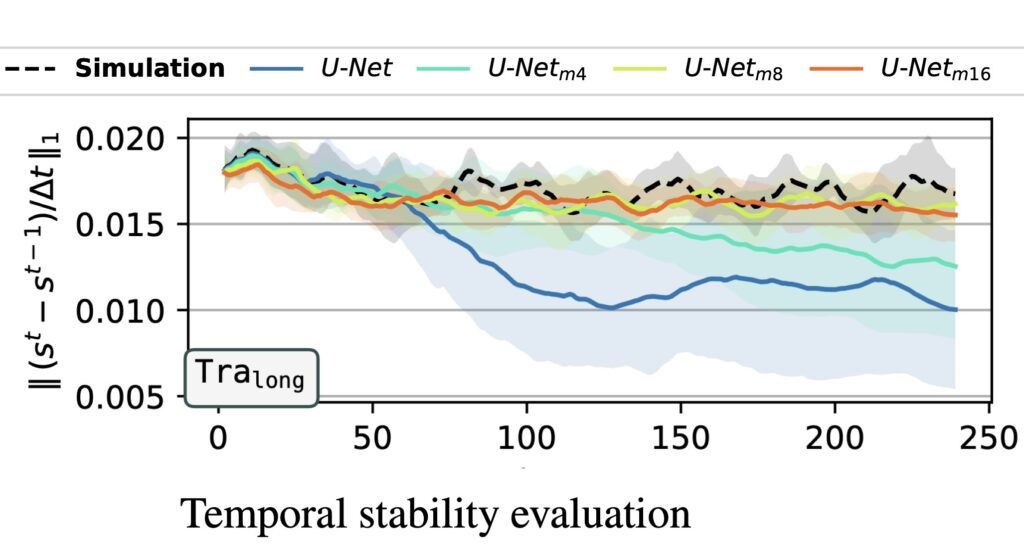
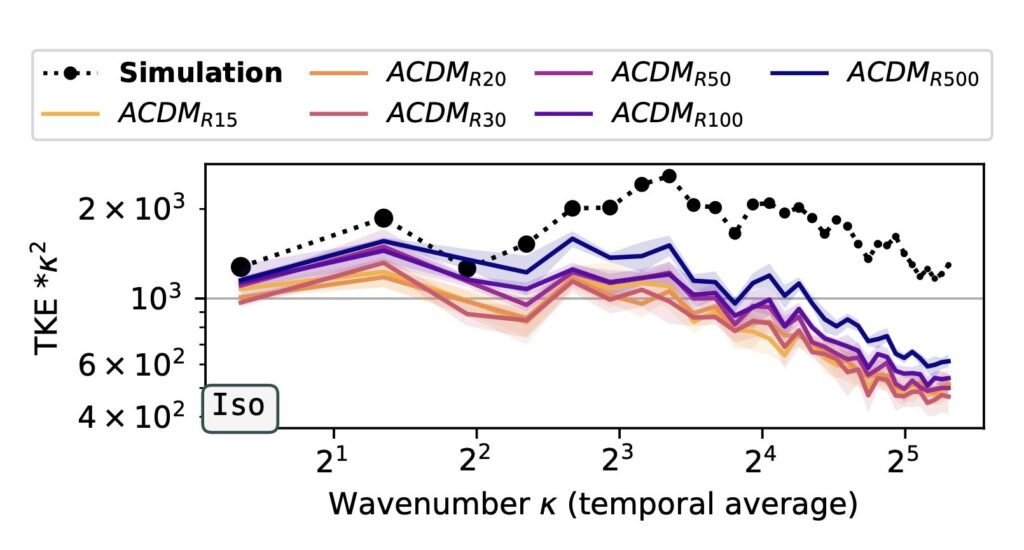
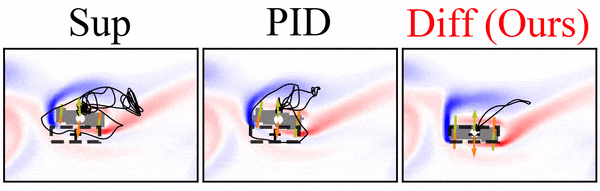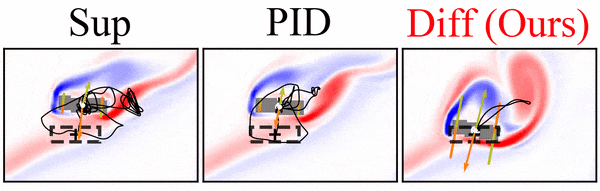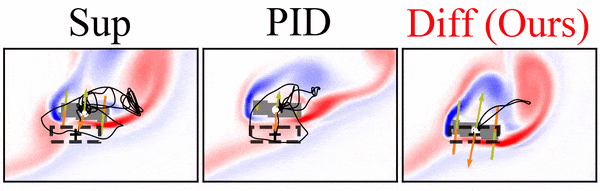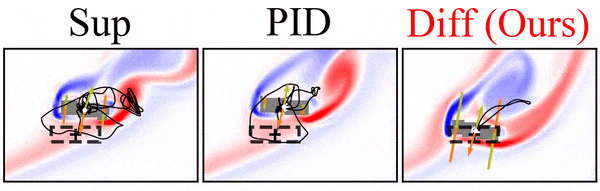
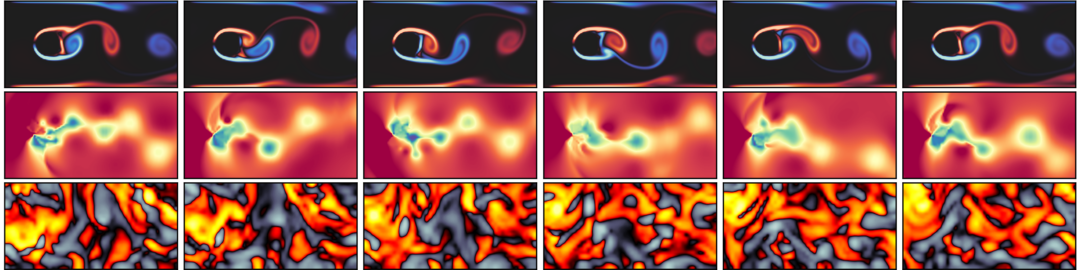
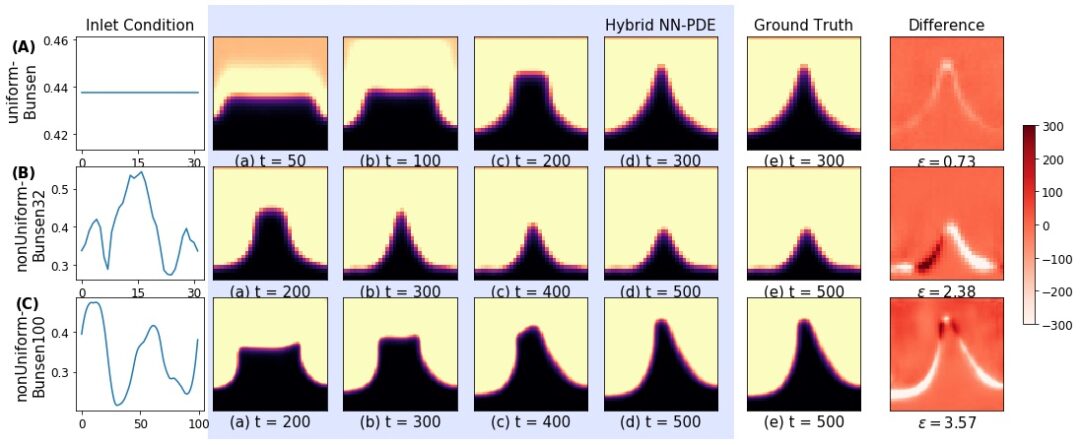
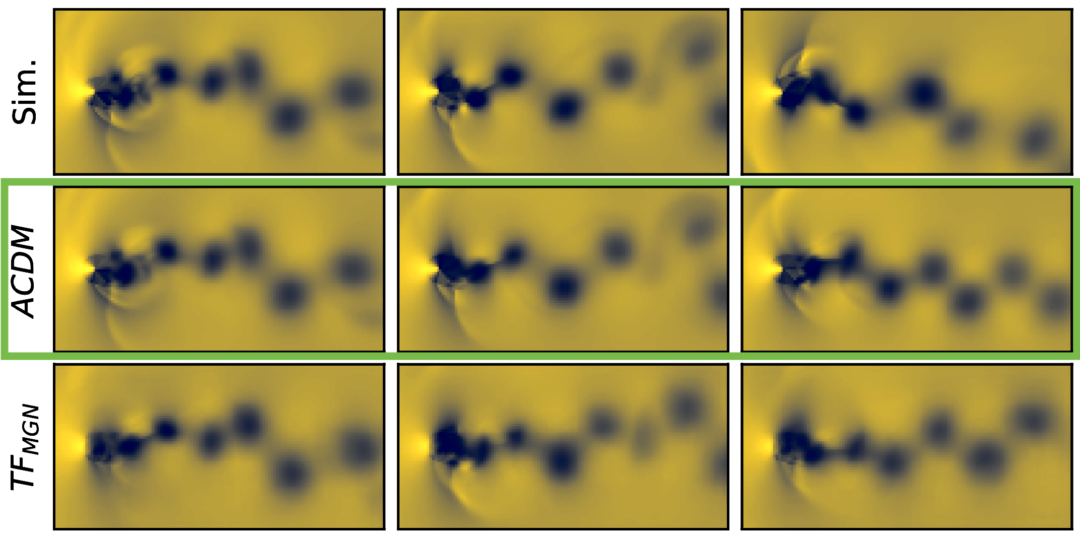
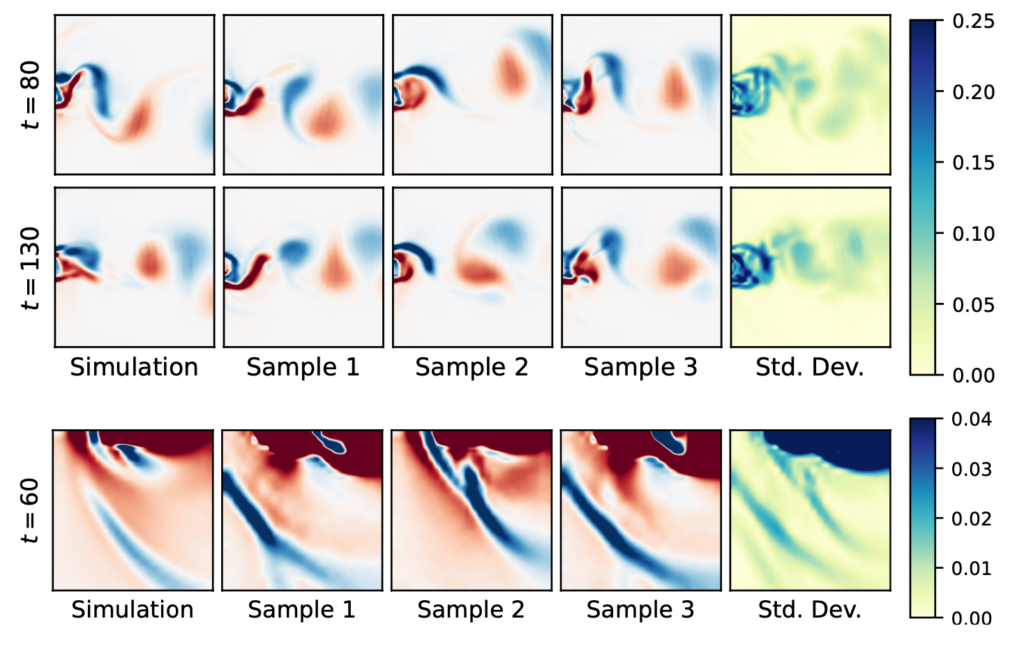
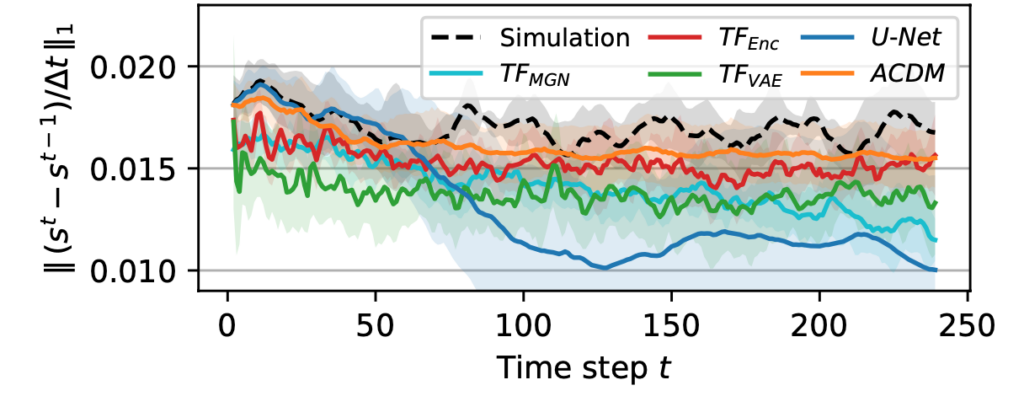
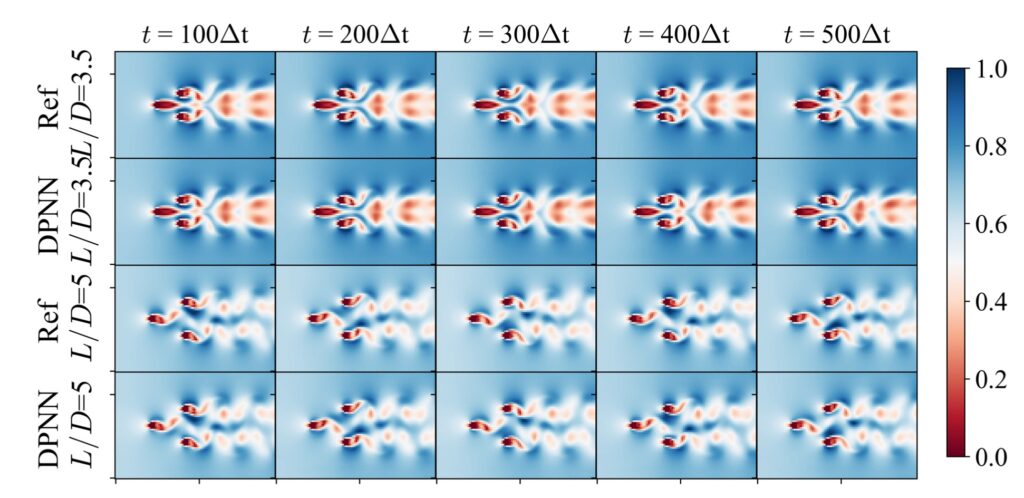
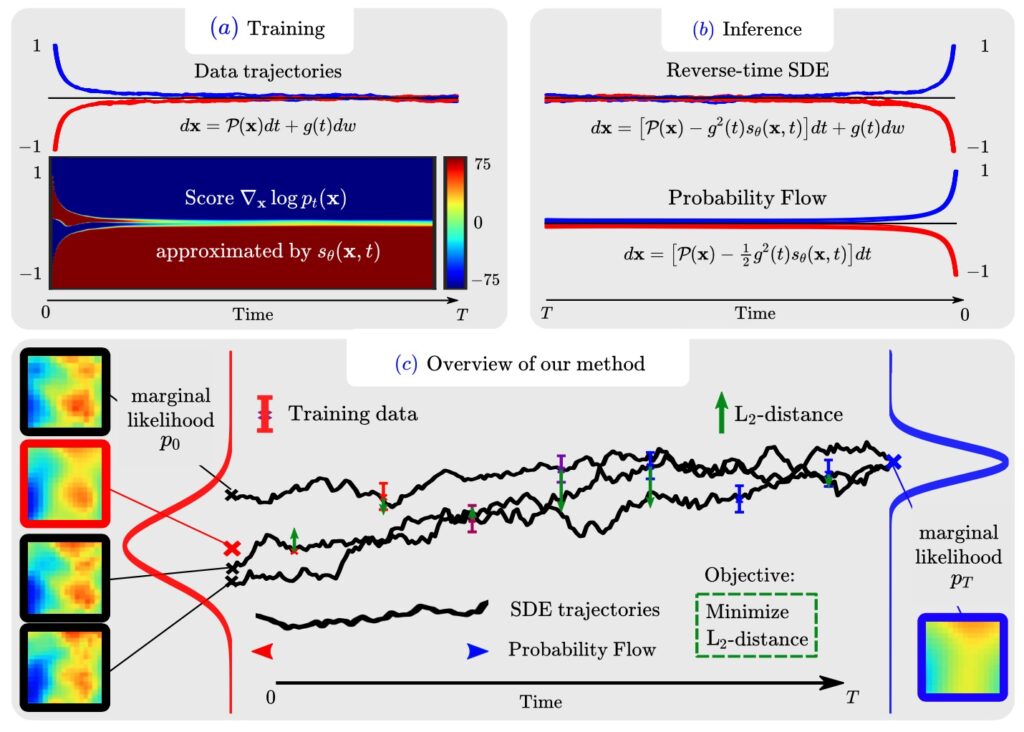
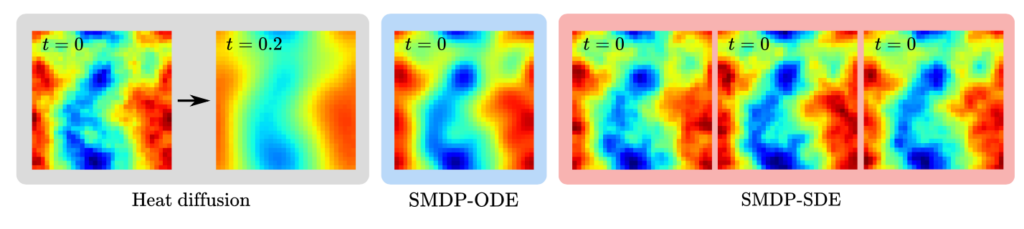
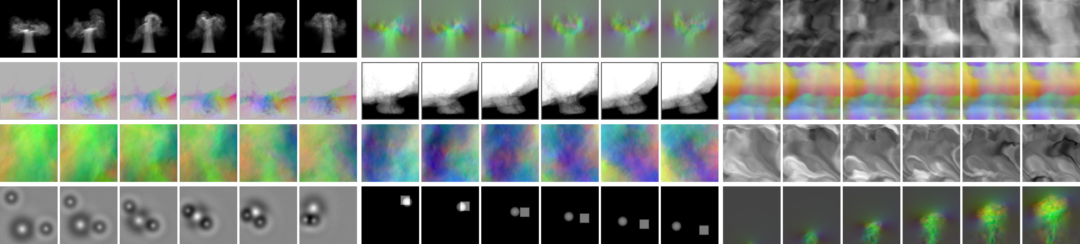
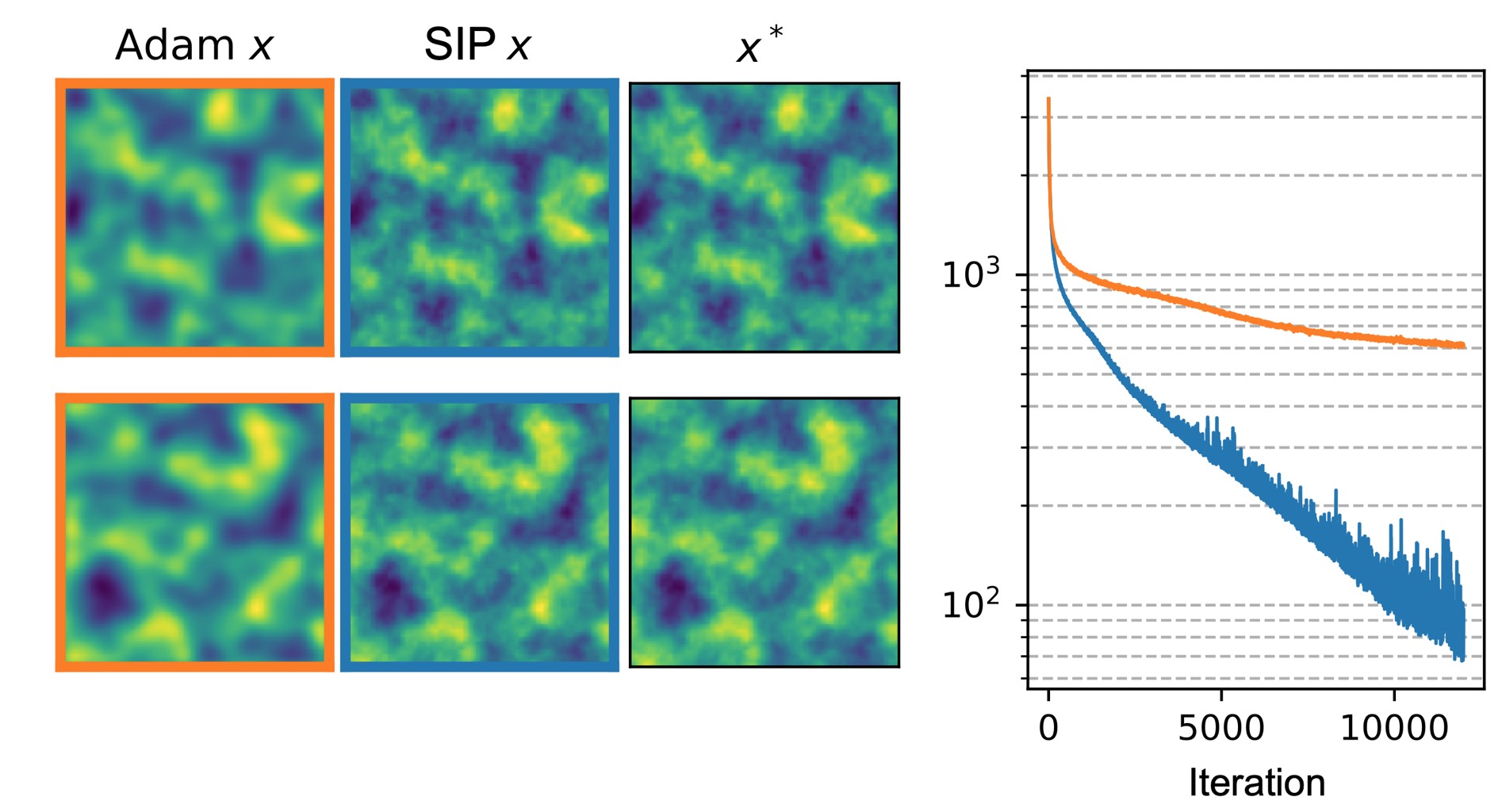
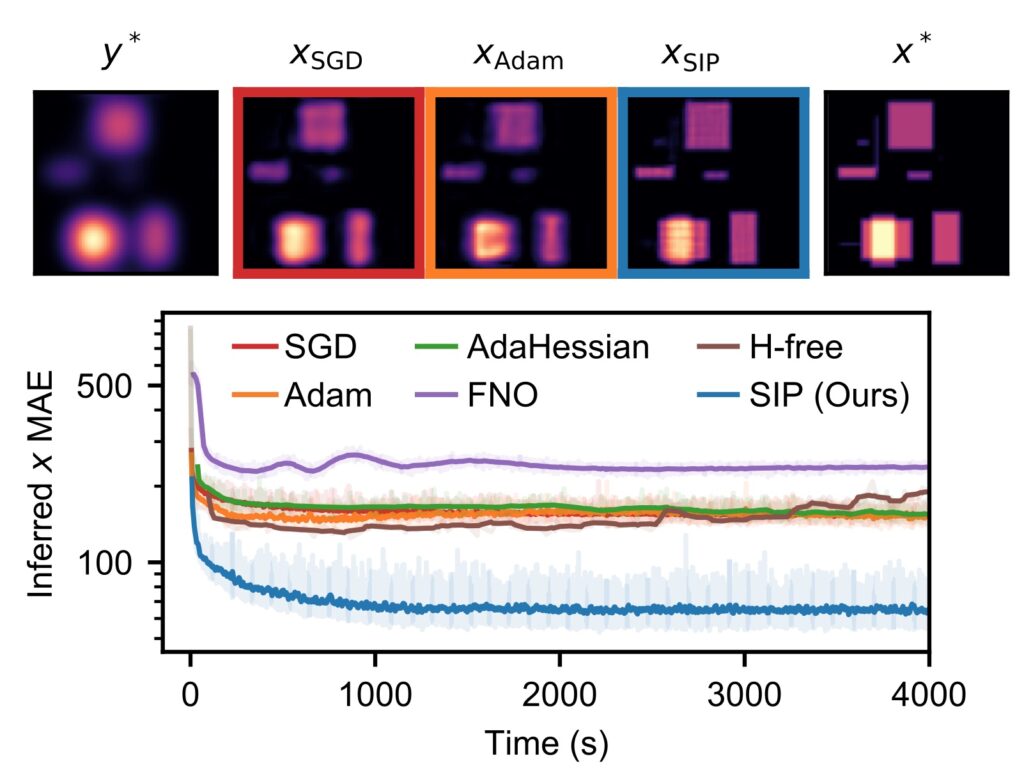
Our paper & source code on using diffusion models to infer RANS solutions for flows around airfoils is online now. It shows that diffusion models finally provide a reliable way to learn full distributions of solutions!
PDF: https://arxiv.org/abs/2312.05320
Source-code: https://github.com/tum-pbs/Diffusion-based-Flow-Prediction/
Here’s an example result, shown in terms of the standard deviation over 100 samples given one set of initial free stream conditions and a fixed airfoil shape:
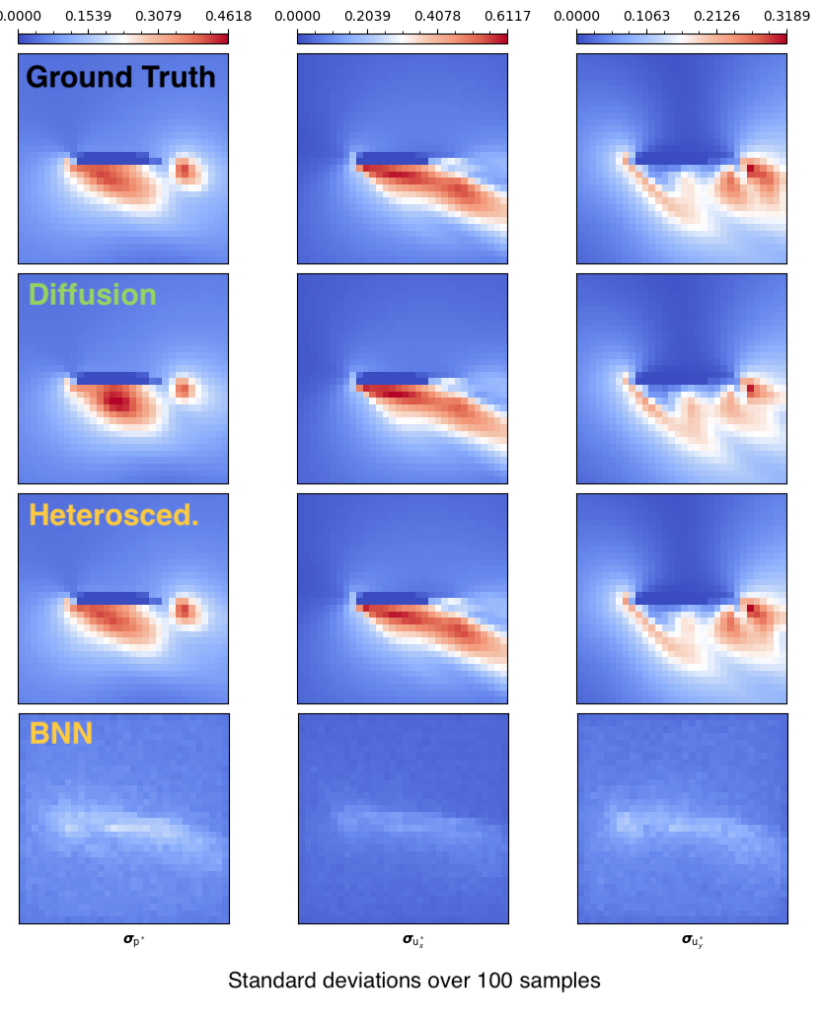
Footnote: the heteroscedastic version (in blue) is not a competitor, it learns mean and standard deviation well, but can’t produce samples.
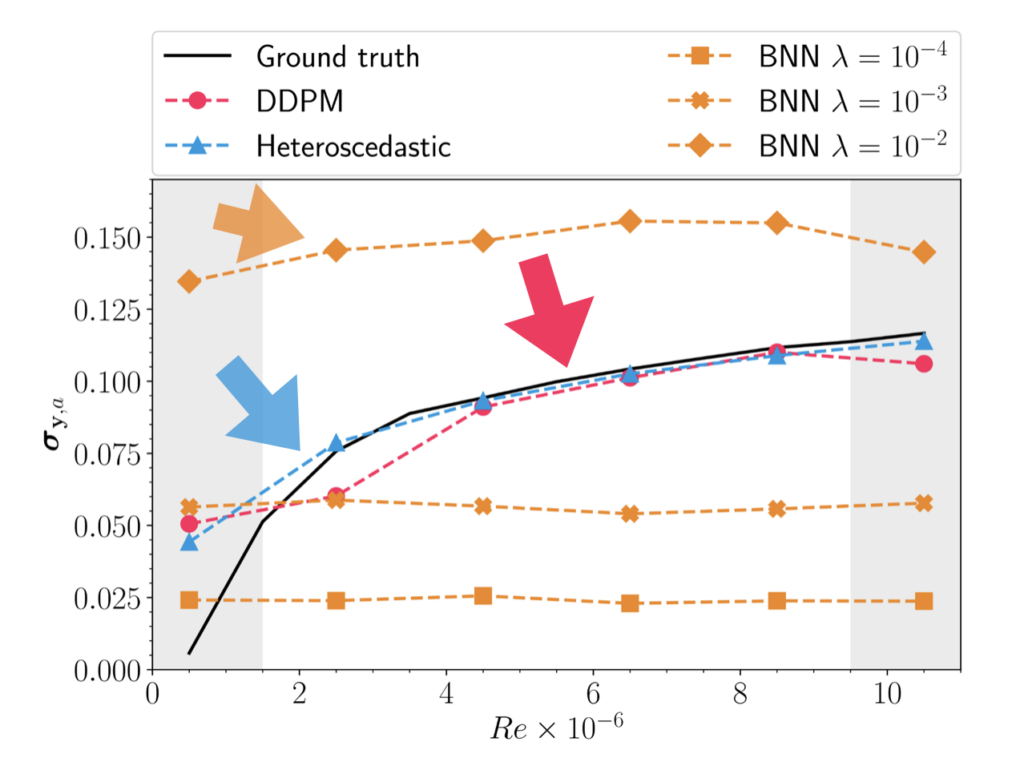
Here’s the full paper abstract for completeness: Leveraging neural networks as surrogate models for turbulence simulation is a topic of growing interest. At the same time, embodying the inherent uncertainty of simulations in the predictions of surrogate models remains very challenging. The present study makes a first attempt to use denoising diffusion probabilistic models (DDPMs) to train an uncertainty-aware surrogate model for turbulence simulations. Due to its prevalence, the simulation of flows around airfoils with various shapes, Reynolds numbers, and angles of attack is chosen as the learning objective. Our results show that DDPMs can successfully capture the whole distribution of solutions and, as a consequence, accurately estimate the uncertainty of the simulations. The performance of DDPMs is also compared with varying baselines in the form of Bayesian neural networks and heteroscedastic models. Experiments demonstrate that DDPMs outperform the other methods regarding a variety of accuracy metrics. Besides, it offers the advantage of providing access to the complete distributions of uncertainties rather than providing a set of parameters. As such, it can yield realistic and detailed samples from the distribution of solutions.